Weight and mass

Mass is an intrinsic property of matter. Every proton has the same mass. Every neutron has the same mass, slightly more than a proton. Every electron has the same mass, much less than protons or electrons.

The periodic table of the elements contains rich information about atoms. For example, a helium nucleus is made of two protons and two neutrons. The mass is slightly less than the sum of the constituent parts because some matter goes into the binding energy that holds the nucleus together.

An object's mass is effectively a count of how many atoms go into making up the object, since we know the masses of the atoms.

In the most fundamental sense, we really do not know what mass is, only that massive objects exert an attractive force on each other.

The gravitational force between two massive particles is defined as the product of the masses divided by the square of the distance between them. The force is negative because it is attractive. The smaller the distance between the particles, the stronger the force. We use the gravitational constant G to obtain the correct units and also to set the scale relative to other kinds of force like the electromagnetic force. The r-hat unit vector gives the direction of the force.

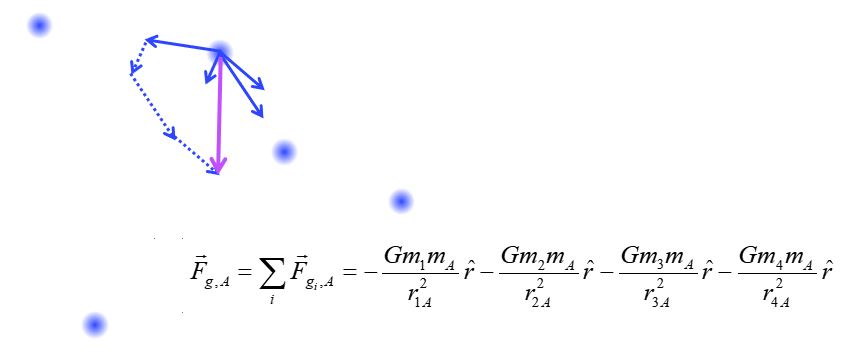
The total gravitational force on a massive particle is the vector sum of all of the forces from other massive particles. As seen in this example, the net gravitational force may not even point in the direction of any one of the particles.

We can also define the gravitational field. It is related to the gravitational force, but it is different in a fundamental way. A field is defined at every point in space. We can define a gravitational field at a point P even if there is no massive particle located there. The gravitational field g is a vector quantity, defined as the net gravitational force divided by mass m. The direction of the field is the direction of the gravitational force that a small test mass would feel if it was placed at P. The gravitational field has units of acceleration.

If we make the assumption that a large massive object like Earth has spherical symmetry, we can treat it as a point mass located at its center.

The gravitational force between a point with mass m and Earth, if the point is near the surface of Earth, is defined using the mass and radius of Earth as shown. We use a lower case g to denote the acceleration due to gravity on a massive particle near the surface of Earth.

Notice that the acceleration of a massive particle does not depend on the mass of the particle.

Weight is the reading given by a spring scale when the object is at rest relative to the scale. In many cases, the scale is at rest with the surface of Earth, so weight is the amount of force generated by the gravitational attraction between two massive objects.

Your mass is the same, whether you are on the Moon or on Earth the number of atoms has not changed. Your weight is very different, because the mass divided by the radius squared is much different for the Earth and Moon.

The value showing in this diagram is 480 kilograms, or the mass of the baby elephant. The weight of the elephant is (480 kg)x(9.8 m/s2) = 4700 N.

The magnitude of the force of a spring depends on how far it is compressed or expanded: F = -kx. The spring constant k is a measure of the stiffness of a spring. We can utilize this to make a spring scale to measure the weight of an object of mass M. The normal force exerted upward on the object of mass M is equal and opposite of the weight. A hanging spring scale works the same, except that it measures the tension pulling down on the spring as the weight.

Weight in an elevator

If we define weight as the reading on a spring scale, a person in an elevator that was accelerating upward would weigh more than they would standing on a scale that was at rest with the surface of Earth, or moving at constant velocity. A person in an elevator that was accelerating downward would with more than mg, and a person in freefall would be weightless.

Practice problems

1. An elevator is suspended by a cable. The elevator is moving upward and slowing down. Which free-body diagram is correct for this situation?

4. A pendant is hanging at rest from two chains, with equal tensions in the chains. Find the tension in a chain. Start by drawing a free-body diagram with the pendant at the origin.

Consider the case where the mass of the pendant is 0.12 kg and the angles  each equal 15° from the  vertical axis. Enter your answer in N.